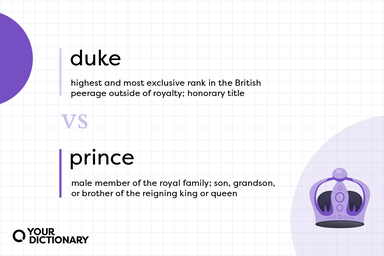
Rank-nullity Theorem Definition
noun
A theorem about linear transformations (or the matrix that represent them) stating that the rank plus the nullity equals the dimension of the entire vector space (which is the linear transformation's domain).
If — for a homogeneous system of linear equations — there are V unknowns and R (linearly independent) equations then, according to the rank-nullity theorem, the solution space is N equals V − R dimensional.
Wiktionary
Find Similar Words
Find similar words to rank-nullity theorem using the buttons below.