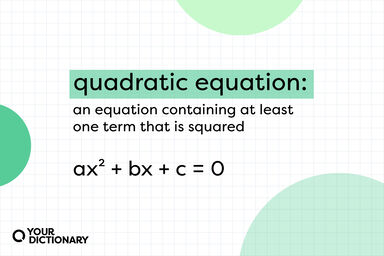Legendre's Differential Equation Definition

The equation that defines the Legendre polynomials: {d \over dx} \left (1-x^2) {d \over dx} P_n(x) \right + n(n+1)P_n(x) = 0.

Wiktionary

Origin of Legendre's Differential Equation

  • Named after French mathematician Adrien-Marie Legendre (1752-1833).

    From Wiktionary

Related Articles

Find Similar Words

Find similar words to Legendre's differential equation using the buttons below.

Words Starting With

LLELEG

Words Ending With

NONION

Words Starting With L and Ending With N

Starts With L & Ends With NStarts With LE & Ends With NStarts With L & Ends With ON

Word Length

Words Near Legendre's Differential Equation in the Dictionary