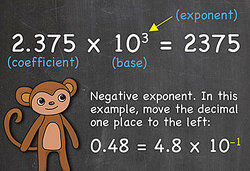
Scientific Notation Examples: Shortening Equations & Numbers

In scientific notation:

• The first number in the mathematical equation is called the "coefficient." The coefficient must be greater than or equal to one and less than 10. For example to create the scientific notation for the number 256, the coefficient would be 2.56.
• The second number in the equation is a power of 10, written as 10 with an exponent, like 10² which stands for 10 x 10.

Combining these two numbers would create this scientific notation equation for 256 - 2.56 x 10².

A negative exponent shows the decimal is moved that many places to the left and a positive exponent shows the decimal is moved that many places to the right.

Here are examples of scientific notation:

• 1 = 10⁰

• 10 = 1 x 10¹

• 100 = 1 x 10²

• 1000 = 1 x 10³

• 10000 = 1 x 10⁴

• 100000 = 1 x 10⁵

• 42,000,000 = 4.2 x 10⁷

• 676,000,000,000 = 6.76 x 10¹¹

• 0.1 = 1 x 10^-1

• 0.01 = 1 x 10^-2

• 0.001 = 1 x 10^-3

• 0.0001 = 1 x 10^-4

• 0.00001 = 1 x 10^-5

• 0.00000078 = 7.8 x 10^-7

• 0.000000000000000 = 3.56 x 10^-13

Explore how scientific notation is used to express large numbers.

• 7 x 10⁹ = Population of the world is around 7 billion written out as 7,000,000,000

• 1.08 x 10⁹ = Approximate speed of light is 1080 million km per hour or 1,080,000,000 km per hour

• 2.4 x 10⁵ = Distance from the Earth to the moon is 240 thousand miles or 240,000 miles

• 9.3 x 10⁷ = Distance from the Earth to the sun is 93 million miles or 93,000,000 miles

• 3.99 x 10¹³ = Distance from the sun to the nearest star (Proxima Centauri) is 39,900,000,000,000 km

• 9.4605284 × 10¹⁵ = Distance light travels in a year is less than 9.5 trillion kilometers, or exactly 9,460,528,400,000,000 km

• 1.4 x 10⁸ = Amount of water surface area on the Earth is 140 million square miles or 140,000,000 square miles

• 1.0 x 10¹⁴ = Approximate number of cells in the human body is 100 trillion or 100,000,000,000,000

• 1.332 x 10^-3 = Density of oxygen is 1332 millionths g per cc or .001332 g per cc
• 2.4 x 10^-3 = Diameter of a grain of sand is 24 ten-thousandths inch or .0024 inch
  

• 7.53 x 10^-10 = Mass of a dust particle is 0.000000000753 kg

• 9.1093822 × 10^-31 = Mass of an electron is 0.00000000000000000000000000000091093822 kg

• 4.0 x 10^-7 = Length of the shortest wavelength of visible light (violet) is 0.0000004 meter

Scientific notation can make it easier to perform mathematical functions with large numbers.

Here are five examples:

1. Multiply (4 x 10⁴) and (7 x 10⁵)
   1. First 4 x 7 = 28
   2. Next add exponents, 4 + 5 = 9
   3. Result is 28 x 10⁹
   4. Rewrite in standard form, 2.8 x 10¹⁰
2. Divide (6 x 10⁵) by (4 x 10⁴)
   1. 6/4 = 1.5
   2. Subtract exponents 5 - 4 = 1
   3. Answer 1.5 x 10¹ or 15
3. Multiply (4 x 10^-7) and (3.25 x 10⁹)
   1. 4 x 3.25 = 13
   2. Add exponents = -7 + 9 = 2
   3. Answer is 13 x 10² or 1300

Adding and subtraction requires that the exponents be the same, so some manipulation is needed:

1. (2.456 x 10⁵)+ (6.0034 × 10⁸)
   1. Change 2.456 x 10⁵ to 0.002456 x 10⁸
   2. 0.002456 + 6.0034 = 6.005856
   3. Answer is 6.005856 × 10⁸
2. Subtract (7 × 10⁵) - (5.2 ×10⁴)
   1. Change to 7 × 10⁵ to 0.52 × 10⁴
   2. 7 - 0.52 = 6.48
   3. Answer = 6.48 x 10⁴

Now you see lots of examples of scientific notation.